[wpfilebase tag=file id=108 tpl=supplement /]
In Section 2.1.3, we categorized waves by the relationship between the direction of the medium’s movement and the direction of the wave’s propagation. Another useful way to categorize waves is by their shape – square, sawtooth, and triangle, for example. These waves are easily described in mathematical terms and can be constructed artificially by adding certain harmonic frequency components in the right proportions. You may encounter square, sawtooth, and triangle waves in your work with software synthesizers. Although these waves are non-sinusoidal – i.e., they don’t take the shape of a perfect sine wave – they still can be manipulated and played as sound waves, and they’re useful in simulating the sounds of musical instruments.
A square wave rises and falls regularly between two levels (Figure 2.20, left). A sawtooth wave rises and falls at an angle, like the teeth of a saw (Figure 2.20, center). A triangle wave rises and falls in a slope in the shape of a triangle (Figure 2.20, right). Square waves create a hollow sound that can be adapted to resemble wind instruments. Sawtooth waves can be the basis for the synthesis of violin sounds. A triangle wave sounds very similar to a perfect sine wave, but with more body and depth, making it suitable for simulating a flute or trumpet. The suitability of these waves to simulate particular instruments varies according to the ways in which they are modulated and combined.
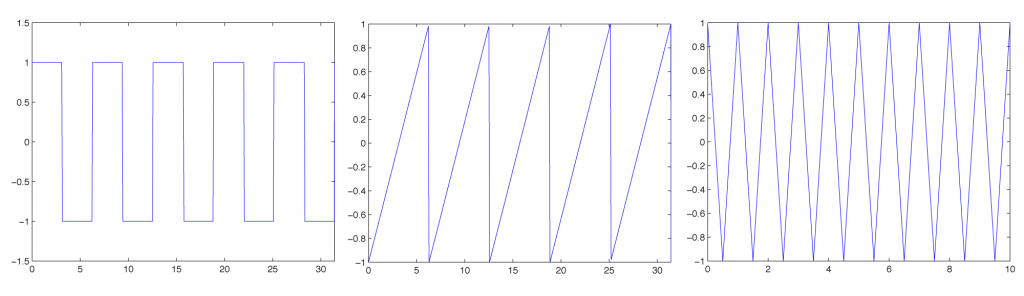
[aside]If you add the even numbered frequencies, you still get a sawtooth wave, but with double the frequency compared to the sawtooth wave with all frequency components.[/aside]
[wpfilebase tag=file id=11 tpl=supplement /]
Non-sinusoidal waves can be generated by computer-based tools – for example, Reason or Logic, which have built-in synthesizers for simulating musical instruments. Mathematically, non-sinusoidal waveforms are constructed by adding or subtracting harmonic frequencies in various patterns. A perfect square wave, for example, is formed by adding all the odd-numbered harmonics of a given fundamental frequency, with the amplitudes of these harmonics diminishing as their frequencies increase. The odd-numbered harmonics are those with frequency fn where f is the fundamental frequency and n is a positive odd integer. A sawtooth wave is formed by adding all harmonic frequencies related to a fundamental, with the amplitude of each frequency component diminishing as the frequency increases. If you would like to look at the mathematics of non-sinusoidal waves more closely, see Section 2.3.2.
[separator top=”1″ bottom=”0″ style=”none”]